Triangle Inequality

The triangle inequality may refer to one of many different theorems, all of which are similar to, derived from or generalisations of the following observation about triangles.

Given a triangle with side lengths $a$, $b$, and $c$, for any choice of the labelling of the sides, $a+b\ge c$. Note that the potential equality is only for the degenerate case, where the vertices are collinear.

In a Normed Vector Space

In a normed vector space, the triangle inequality is an explicit requirement for a vector space norm, and is stated as:

Intuition in ${\mathbb{R}}^2$

In ${\mathbb{R}}^2$, the triangle inequality for the norm induced by the dot product has a clear geometric intuition that aligns with the case of a triangle described at the top of this note.

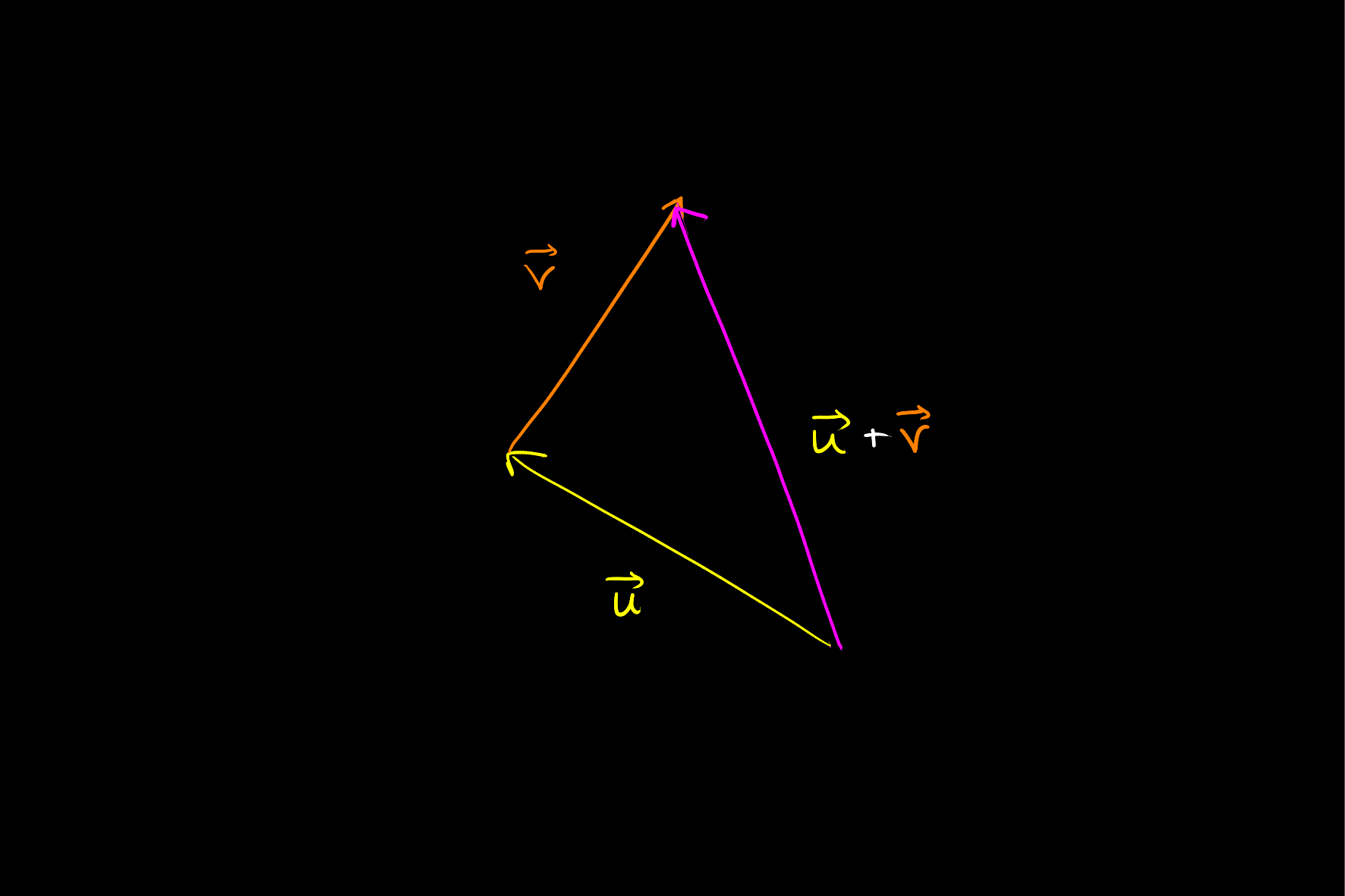
In the Context of Complex Numbers

In the context of complex numbers, the triangle inequality generally refers to:

for $z,w\in \mathbb{C}$.

The geometric intuition in the complex plane is basically identical to that of the triangle inequality for norms in ${\mathbb{R}}^2$.

In a Metric Space

Within a metric space, the triangle inequality, which is one of the requirements for a metric, is that:

Similarly to the case of the norm, in ${\mathbb{R}}^2$ this geometrically represents how the length of one side of a triangle is less than or equal to the sum of the other two.